Хаотические системы связи с модуляцией сигнала на основе управляемой симметрии полуявных конечно-разностных моделей
https://doi.org/10.31854/1813-324X-2024-10-1-6-16
EDN: PRUEAO
Аннотация
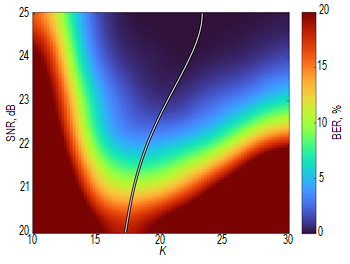
Статья посвящена разработке модели когерентной хаотической системы связи, реализующей новый способ модуляции сигнала на основе управляемой симметрии конечно-разностных схем с последующим экспериментальным анализом эффективности различных методов модуляции. Целью работы является проектирование и исследование имитационной компьютерной модели хаотической системы связи с модуляцией сигнала на основе управляемой симметрии полуявных конечно-разностных схем. Элементами научной новизны обладают конечно-разностные модели приемников/передатчиков, позволяющие реализовать новый способ модуляции хаотических сигналов. Результат: получение имитационной модели когерентных хаотических систем связи с инструментами анализа скрытности и помехоустойчивости. Практическая значимость: имитационная модель хаотической системы связи является необходимым инструментом для анализа работоспособности системы перед ее физической реализацией.
Ключевые слова
нелинейная динамика,
конечно-разностная модель,
полуявные методы интегрирования,
хаотические системы связи
При поддержке: Работа выполнена при поддержке Российского научного фонда (проект № 10-79-10334)
Об авторе
В. Г. Рыбин
Санкт-Петербургский государственный электротехнический университет «ЛЭТИ» им. В.И. Ульянова (Ленина)
Россия
Россия
ассистент кафедры систем автоматизированного проектирования Санкт-Петербургского государственного электротехнического университета «ЛЭТИ» им. В.И. Ульянова (Ленина)
Список литературы
1. Ostrovskii V.Y., Karimov A.I., Rybin V.G., Kopets E.E., Butusov D.N. Comparing the Finite-Difference Schemes in the Simulation of Shunted Josephson Junctions // Proceedings of the 23rd Conference of Open Innovations Association (FRUCT, Bologna, Italy, 13‒16 November 2018). IEEE, 2018. PP. 300–305. DOI:10.23919/FRUCT.2018.8588028
2. Kaddoum G. Wireless Chaos-Based Communication Systems: A Comprehensive Survey // IEEE Access. 2016. Vol. 4. PP. 2621‒2648. DOI:10.1109/ACCESS.2016.2572730
3. Ostrovskii V.Y., Rybin V.G., Karimov A.I., Butusov D.N. Inducing multistability in discrete chaotic systems using numerical integration with variable symmetry // Chaos, Solitons & Fractals. 2022. Vol. 165. Part 1. P. 112794. DOI:10.1016/j.chaos.2022.112794
4. Rahman Z.A.S., Jasim B.H., Al-Yasir Y.I., Hu Y.F., Abd-Alhameed R.A., Alhasnawi B.N. A New Fractional-Order Chaotic System with its Analysis, Synchronization, and Circuit Realization for Secure Communication Applications // Mathematics. 2021. Vol. 9. Iss. 20. P. 2593. DOI:10.3390/math9202593
5. Benkouider K., Bouden T., Sambas A., Mohamed M.A., Sulaiman I. M., Mamat M., et al. Dynamics, Control and Secure Transmission Electronic Circuit Implementation of a New 3D Chaotic System in Comparison With 50 Reported Systems // IEEE Access. 2021. Vol. 9. PP. 152150‒152168. DOI:10.1109/ACCESS.2021.3126655
6. Gokyildirim A., Kocamaz U.E., Uyaroglu Y., Calgan H. A novel five-term 3D chaotic system with cubic nonlinearity and its microcontroller-based secure communication implementation // AEU ‒ International Journal of Electronics and Communications. 2023. Vol. 160. P. 154497. DOI:10.1016/j.aeue.2022.154497
7. Babajans R., Cirjulina D., Capligins F., Kolosovs D., Grizans J., Litvinenko A. Performance Analysis of Vilnius Chaos Oscillator-Based Digital Data Transmission Systems for IoT // Electronics. 2023. Vol. 12. Iss. 3. P. 709. DOI:10.3390/electronics12030709
8. Cirjulina D., Babajans R., Kolosovs D., Litvinenko A. Experimental Study on Frequency Modulated Chaos Shift Keying Communication System // Proceedings of the Workshop on Microwave Theory and Techniques in Wireless Communications (MTTW, Riga, Latvia, 05‒07 October 2022). IEEE, 2022. DOI:10.1109/MTTW56973.2022.9942593
9. Cui S., Zhang J. Chaotic Secure Communication Based on Single Feedback Phase Modulation and Channel Transmission // IEEE Photonics Journal. 2019. Vol. 11. Iss. 5. P. 7905208. DOI:10.1109/JPHOT.2019.2931615
10. Butusov D.N., Karimov A.I., Tutueva A.V. Symmetric extrapolation solvers for ordinary differential equations // Proceedings of NW Russia Young Researchers in Electrical and Electronic Engineering Conference (EIConRusNW, St. Petersburg, Russia, 02‒03 February 2016). IEEE, 2016. PP. 162‒167. DOI:10.1109/EIConRusNW.2016.7448145
11. Voroshilova A., Wafubwa J. Discrete Competitive Lotka–Volterra Model with Controllable Phase Volume // Systems. 2020. Vol. 8. Iss. 2. P. 17. DOI:10.3390/systems8020017
12. Butusov D.N., Ostrovskii V.Y., Karimov A.I., Andreev V.S. Semi-Explicit Composition Methods in Memcapacitor Circuit Simulation // International Journal of Embedded and Real-Time Communication Systems (IJERTCS). 2019. Vol. 10. Iss. 2. PP. 37–52. DOI:10.4018/IJERTCS.2019040103
13. Terentev A.A., Butusov D.N., Fedoseev P.S. Novel Composition ODE Solver based on Semi-Implicit Integration // Proceedings of the International Conference on Soft Computing and Measurements (SCM, St. Petersburg, Russia, 27‒29 May 2020). IEEE, 2020. PP. 128–132. DOI:10.1109/SCM50615.2020.9198821
14. Reich S. Linearly implicit time stepping methods for numerical weather prediction // BIT Numerical Mathematics. 2006. Vol. 46. PP. 607–616. DOI:10.1007/s10543-006-0065-0
15. Tutueva A., Butusov D. Avoiding Dynamical Degradation in Computer Simulation of chaotic Systems Using Semi-Explicit Integration: Rössler Oscillator Case // Fractal and Fractional. 2021. Vol. 5. Iss. 4. P. 214. DOI:10.3390/fractalfract5040214
16. Karimov T., Rybin V., Kolev G., Rodionova E., Butusov D. Chaotic Communication System with Symmetry-Based Modulation // Applied Sciences. 2021. Vol. 11. Iss. 8. P. 3698. DOI:10.3390/app11083698
17. Tutueva A.V., Karimov T.I., Andreev V.S., Zubarev A.V., Rodionova E.A., Butusov D.N. Synchronization of Chaotic Systems via Adaptive Control of Symmetry Coefficient in Semi-Implicit Models // Proceedings of the Ural Smart Energy Conference (USEC, Ekaterinburg, Russia, 13‒15 November 2020). IEEE, 2020. PP. 143–146. DOI:10.1109/USEC50097.2020.9281181
18. Singh P.P. A Novel Chaotic System with Wide Spectrum, its Synchronization, Circuit Design and Application to Secure Communication // Indian Journal of Science and Technology. 2021. Vol. 14. Iss. 28. PP. 2351‒2367. DOI:10.17485/ijst/v14i28.1035
19. Rybin V., Kolev G., Kopets E., Dautov A., Karimov A., Karimov T. Optimal Synchronization Parameters for Variable Symmetry Discrete Models of Chaotic Systems // Proceedings of the 11th Mediterranean Conference on Embedded Computing (MECO, Budva, Montenegro, 07‒10 June 2022). IEEE, 2022. DOI:10.1109/MECO55406.2022.9797125
20. Rybin V., Butusov D., Rodionova E., Karimov T., Ostrovskii V., Tutueva A. Discovering Chaos-Based Communications by Recurrence Quantification and Quantified Return Map Analyses // International Journal of Bifurcation and Chaos. 2022. Vol. 32. Iss. 9. P. 2250136. DOI:10.1142/S021812742250136X
21. Pérez G., Cerdeira H.A. Extracting messages masked by chaos // Physical Review Letters. 1995. Vol. 74. Iss. 11. P. 1970. DOI:10.1103/PhysRevLett.74.1970
22. Li S., Chen G., Alvarez G. Return-map cryptanalysis revisited // International Journal of Bifurcation and Chaos. 2006. Vol. 16. Iss. 5. PP. 1557–1568. DOI:10.1142/S0218127406015507
23. Yang T., Yang L.B., Yang C.M. Cryptanalyzing chaotic secure communications using return maps // Physics Letters A. 1998. Vol. 245. Iss. 6. PP. 495–510. DOI:10.1016/S0375-9601(98)00425-3
Рецензия
Для цитирования:
Рыбин В.Г. Хаотические системы связи с модуляцией сигнала на основе управляемой симметрии полуявных конечно-разностных моделей. Труды учебных заведений связи. 2024;10(1):6-16. https://doi.org/10.31854/1813-324X-2024-10-1-6-16. EDN: PRUEAO
For citation:
Rybin V. Chaotic Communication Systems with Signal Modulation Based on Controlled Symmetry of Semi-Implicit Finite-Difference Models. Proceedings of Telecommunication Universities. 2024;10(1):6-16. (In Russ.) https://doi.org/10.31854/1813-324X-2024-10-1-6-16. EDN: PRUEAO
Просмотров:
431
Послать статью по эл. почте 